|
|||||||||||||
"
In a certain sense, everything is everywhere at all times. For every location involves an aspect of itself in every other location. Thus every spatio-temporal standpoint mirrors the world."—Ralph Waldo Emerson Compensation
Chapter 7
From The Book:
Everything Forever:
Learning to See Timelessness
"
…it is the nature of synchronicity to have meaning and, in particular, to be associated with a profound activation of energy deep within the psyche. It is as if the formation of patterns in the unconscious mind is accompanied by physical patterns in the outer world."—F. David Peat
|
From
Chapter 7
Einstein once spoke of the physicist David Bohm as his successor. Bohm introduced concepts of Implicate Order and Explicate Order. Bohm defined explicate order as the order of the physical world. He defined implicate order as the source of explicate order, and as an underlying whole that physical form constantly unfolds out from and enfolds back into. Others had constructed related models of two orders, including Henri Bergson and William Yeats in the early 1900's. Bohm meant his concepts to replace order and disorder although he was not able to convince other scientists of this necessity, and Bohm struggled with depression at not being able to convince the scientific community of the scientific value of his discoveries. In describing Implicate Order Bohm writes:
The two directions of order are probably the most important features of nature that we will ever understand. There are endless applications of two orders in every field, in physics, biology, geology, politics, and psychology, just naming the more obvious fields. The theory of two orders generally describes all change, all patterns, all definitive form, so what if anything could be excluded. As we learn to perceive the cosmos as one type of order transforming into another, doors of comprehension will open beyond our wildest dreams. In order to understand how the whole can exist in every part, Bohm became interested in the mechanics of holographic photography. Using lasers, a holographic image is recorded evenly across the photographic film. Consequently any region of the film contains information about the whole image, so any small region of the film can recreate the image, although in poorer resolution than the entire film produces. Bohm believed all matter is unfolded out of what he eventually described as a holomovement, which meant that matter could also enfold and so return into the holomovement. Bohm considered quantum mechanics to be a process of unfolding and enfolding. He imagined the universe as an infinite sea of space and energy out of which matter could be unfolded, which he called explicating, and enfolded which he called implicating, which, in Bohm’s words, “together are a flowing, undivided wholeness. Every part of the universe is related to every other part but in different degrees.” In an interview published in Omni magazine conducted by the physicist F. David Peat and John Briggs, Bohm explained his concept of enfoldment:
The process of unfolding and enfolding suggests a whole exists primarily as a base, and although Bohm described the whole as being dynamic and in constant motion in most of his writings, in his later years he began to describe time as occurring within timelessness. In hindsight it appears that Bohm didn’t focus enough on the timeless nature of patterns. He didn’t recognize consciously enough that ordinary patterns reveal the implicate order, although in Bohm’s analogies you find the same type of examples that I use to explain the transition from grouping to symmetry order. One of Bohm’s favorite analogies spawned from a scientific program he’d seen on a television show. The program featured a small scientific wonder, where an insoluble drop of dark ink in one process disappears uniformly into a glycerin, then in the opposite process the drop reappears.
Of course we can accomplish this same transformation less dramatically with any container filled with water and oil, and simply shake the container until the two liquids mix together evenly. Then at rest electrostatic cohesion and gravity will rather quickly re-separate the liquids into two pure groups. What these analogies of mixing and separation attempt to convey is that material form can integrate and disappear into a whole. This suggests an equivalency between matter and that invisible background, a background which we know simply to be space. All the empty space in the universe, which we naturally assume to be less than the physical matter we are able to interact with, is actually more full of content than the surface of form we see due to light waves. What we imagine to be empty space contains the whole of everything. It is a considerably different way of looking at the world, but the message is that matter is constantly unfolding out of and refolding into a larger balanced whole. Bohm writes:
In the book, The Holographic Universe the writer Michael Talbot integrated Bohm’s vision of two kinds of order with the work of Karl Pribram, a famed neurophysiologist who also became interested in holographic photography as a means of explaining the mysteries of human memory. Talbot also suggests the whole universe is a hologram. He writes:
Unfortunately, the mainstream of other scientists didn’t catch on to Bohm’s vision of two kinds of order, and he struggled with despair and depression at not being able to convince the scientific community of the scientific value of an enfolded order, a struggle I inherited. Without knowing I was dramatically influenced, I encountered Bohm’s concept of implicate order many years before I discovered and learned to present my own system of seeing the same thing, the same principles, the same process in nature. Then rediscovering and studying Bohm’s work in greater detail, I now realize my practical grouping order and symmetry order concepts are simply an extension of Bohm’s concepts. I now believe the only reason Bohm’s visionary accomplishments were kept from having greater impact is that he was led more to challenge how quantum mechanics was being interpreted in his day rather than led to explore how the terms order and disorder are defined, as well as the second law’s usage of the terms order and disorder. Also I think he tried to convince the wrong audience. Judging from my own experiences I am sure it would have taken years for even a fully convincing challenge to sink in to the sometimes dull collective mind of science, which grounded in the present dry paradigm tends to shy away from profound concepts altogether. One of the most important lessons I convey perhaps uniquely from Bohm, is that symmetry order is an entirely separate component of the patterns we find in nature, so that we can see the unfolding and enfolding process as a governing system, visible not only within the general evolution of time, but also present within each individual, and within humanity as a whole. I expect anyone who reads this book will eventually be able to observe any pattern in their world and recognize the two components of order not only contributing to the appearance of order, but also the appearance of what we think of as disorder. The Absence of One Order Creates the Other Both the Irish poet William Yeats, who received the Nobel Prize for literature in 1923, and the French philosopher Henri Bergson who received a Nobel in literature in 1927, wrote of two fundamental orders at work in the evolutionary process of the universe. Although the nature of the orders was not described as clearly as Bohm managed with his implicate and explicate concepts, both men recognized the exclusive quality of the two orders more clearly than Bohm. In his book entitled Creative Evolution Bergson writes:
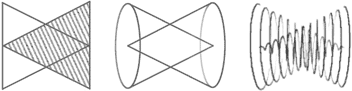
Anywhere that a substance gravitates together, grouping order has increased while symmetry order has decreased. Anyplace where things spread more evenly throughout any frame of reference, symmetry order has increased while grouping order has decreased. Generally, one type of order cannot increase without decreasing the other. Each order has its own direction. Each order is unique. And all there is throughout the universe is order. We observe order in the universe and are amiss at why it exists over disorder. We typically imagine that our corner of the universe must be a tiny island of order within a greater chaos. The term order within chaos is common. However, once we learn to appreciate the two kinds of order, existentially speaking, it seems more apt to say that chaos exists within order. If the disorder of symmetry is inversely the order of grouping, and if every pattern consists of a combination of two orders, then all there can be is order. In the poetic words of the photographer Catherine Ames, “the order and chaos are one.” The Gyres After achieving his Nobel in 1923 the poet William Yeats came to portray the transformation from one order to the other with what he called gyres. These images relate to the double wedge shape of pattern space, but they more accurately portray the measure or intensity of one order compared to the other throughout the entire span of what is possible. They represent how the two orders combine and so become two parts of the same description.
The two cones effectively represent the integration and inseparability of grouping and symmetry orders. It is said that Yeats discovered the images through the mystical experience of his wife Georgie Hyde-Lee, who demonstrated a gift for automatic writing. In various ways Yeats diagrammed two cones or wedges embedded together. Yeats notes that the information his wife acquires mystically seems to come from a common dream they and a few others shared. Noteworthy, it is well known that Yeats studied the writings of Emanuel Swedenborg, a Swedish scientist in his time turned religious leader, who wrote, “All physical reality, the universe as a whole, every solar system, every atom, is a double cone; where there are ‘two poles one opposite the other, these two poles have the form of cones.” The second law is meant to describe the flow of patterns and processes occurring in nature. It attempts to describe the fundamental way that the physical universe changes as time passes, stating that the overall entropy of a system; entropy being the measure of spent energy, always increases with time, which is true, there is no way to get around that law of nature, but the second law also makes a statement which erroneously assumes a connection between a loss of usable energy and an increase in disorder. And we find now that this part of the second law cannot be true, primarily because there is no such thing as general disorder. Symmetry order is the disorder of grouping order, and grouping order is the disorder of symmetry order. If the order of one is the disorder of the other, then there is no room for a general disorder. All there is in nature is ordered patterns of one type or the other, and combinations thereof. We envision order and chaos as being opposed, and chaos theorists speak of an order at the edge of chaos, but neither accurately represents order as it exists in nature. The order that is so visible throughout our universe springs from combinations of imbalance and balance. Whether we speak of the even distribution of galaxies, or gaseous particles that disperse evenly throughout available space, we find what is happening is that time is evolving away from the most extreme state of imbalance to the most extreme state of balance. When one learns to see this transition, it suddenly is visible in everything from red hot flowing materials that solidify into rock or steel, to water vapor which crystallize into a snowflake. My own early diagram, meaning to show the opposition and exclusivity of two orders was simply of a square split in half by opposite corners, and likewise was meant to show that the extreme of one order type is the disorder of the other.
Figure
7.4: Order gradients indicating the absence of a general Symmetry order is responsible for all the qualities of, and preferences for, balance in the world, from spiral galaxies to the dissipation of gases, even perhaps to the human yearning for peace. In regards to the Universe as a whole, the ultimate singularity, and the ultimate example of perfect symmetry or sameness, is plain old empty space. The single property of the whole universe, from which everything is made, and which everything is transforming back into, is just space, although in learning this, it is so important to understand that things are less than, not more than space. The entire world of material things is a fragment of the whole. Everything we know as real is less than the space it exists in, and not more than empty space or nothing. That low opinion we have of nothingness is all wrong. What we think is nothing is perfect symmetry. Presently we have it all backwards.
Physicists and cosmologists have long questioned whether a state of perfect symmetry ever existed in the past, we just
haven't ever considered it as a possible future. We have been convinced instead, because we observe a measure of
randomness that the order of the universe is simply winding down. But if anything it is winding up! Everything is
enfolding together. The final state of zero which the universe has been evolving toward since the very dawn of time
is simply the native state of the Universe. It is the timeless whole. It is truly everything forever. And we aren’t
really becoming, we are already there. The universe we know, the past, the future, and the infinity of other universes,
all exist simultaneously. We are inside that whole. We are a part of the native state of zero, part of the eternal
present. The following essay is an extension of Bohm's concepts into a more practical model of the two orders, in which the two orders are seen to exist physically in the construction of ordinary patterns. This development has led to using new terms, although the underlying natures of definitive form and enfoldment are visibly the same that David Bohm was identifying.
Contents This page last updated May 20th, 2007 |
| Your welcome to send an e-mail and share your thoughts when you're done reading. |
|
Homepage | Part One | Part Two | Part Three | Part Four | Contents | e-mail |
|
|
© Gevin Giorbran, Copyright 1996 - 2007 All rights reserved. Privacy Policy | Usage Policy |
|
|


 The Two Kinds of Order
The Two Kinds of Order Obviously Bergson somehow recognized how any decrease in the measure of one type of order, increases the order of
the other type. This can be recognized if we consider a breakdown in the purity of each order. Suppose we switch
two oppositely colored squares on a checkerboard. The inconsistency in the symmetrical arrangement stands out like
a beacon. The rhythmic order of symmetrical pattern has been broken. The symmetry order is clearly diminished. And
yet the fusing of three colored squares into one larger block is an increase in a type of order. Grouping order has
increased. The greater measure of symmetry order isn’t suddenly destroyed, we are only one step away from an
unbroken perfect lattice, it is merely diminished.
Obviously Bergson somehow recognized how any decrease in the measure of one type of order, increases the order of
the other type. This can be recognized if we consider a breakdown in the purity of each order. Suppose we switch
two oppositely colored squares on a checkerboard. The inconsistency in the symmetrical arrangement stands out like
a beacon. The rhythmic order of symmetrical pattern has been broken. The symmetry order is clearly diminished. And
yet the fusing of three colored squares into one larger block is an increase in a type of order. Grouping order has
increased. The greater measure of symmetry order isn’t suddenly destroyed, we are only one step away from an
unbroken perfect lattice, it is merely diminished.
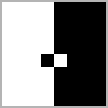 Alternatively, at the other end of order, if we group together the game pieces by colors on each side of the
board, and mistakenly displace one colored piece with another, the diminished purity of the grouping order
stands out vividly here also. The purity of each of the two groups is contaminated, yet note here how this is
a first move toward increased symmetry order. There isn’t actually an increase in any general form of disorder.
Each of these first steps away from an unbroken high order is both an increase of an order and a decrease of an
order. Hence the order of one type is the disorder of the other.
Alternatively, at the other end of order, if we group together the game pieces by colors on each side of the
board, and mistakenly displace one colored piece with another, the diminished purity of the grouping order
stands out vividly here also. The purity of each of the two groups is contaminated, yet note here how this is
a first move toward increased symmetry order. There isn’t actually an increase in any general form of disorder.
Each of these first steps away from an unbroken high order is both an increase of an order and a decrease of an
order. Hence the order of one type is the disorder of the other.